Answer:
The coefficient of
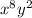 is
is
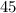 .
.
Explanation:
By the binomial theorem we have that
 .
.
where
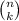 is known has a binomial coefficient and it can be compute by
is known has a binomial coefficient and it can be compute by
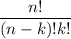 . The symbol
. The symbol
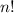 stands for the factorial of
stands for the factorial of
 , that is to say,
, that is to say,

So if you have
 then we can replace on the expression of the binomial theorem to obtain
then we can replace on the expression of the binomial theorem to obtain
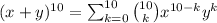
In order to obtain the coefficient of
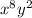 we find the term where
we find the term where
 , that is to say,
, that is to say,
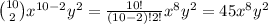 . We conclude that the coefficient we were looking for is
. We conclude that the coefficient we were looking for is
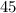 .
.