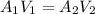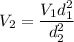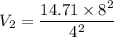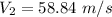Question

Air flows through a circular duct with a diameter of 8 cm at room temperature. The gauge pressure readings for the total pressure and the static pressure are 200 Pa and 70 Pa, respectively. Assume that this system is in a room that is at a pressure of 100,000 Pa and a temperature that is 300K. A. What is the velocity of the air in this 8 cm diameter duct. Give your answer in m/s. B. Furthermore downstream, the diameter of the duct decreases to 4 cm. What is the new velocity of the air when the diameter is 4 cm? Give your answer in m/s.

Answer 1

V=14.71 m/s

`V_2=58.84\ m/s`

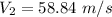
Step-by-step explanation:

Given that

Diameter d= 8 cm

Total pressure = 200 Pa (gauge)

Static pressure = 70 Pa (gauge)

We know that

Total pressure =Static pressure + Dynamic pressure

`Total\ pressure = Static\ pressure +(\rho V^2)/(2)`

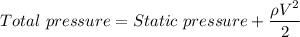
Density of air at room temperature

`\rho = 1.2\ kg/m^3`

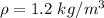
Now by putting the values

`200 = 70 +(1.2* V^2)/(2)`

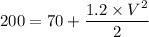
V=14.71 m/s

When diameter is reduced to 4 cm

For same flow rate

We know that

`A_1V_1=A_2V_2`

`V_1d_1^2=V_2d_2^2`

`V_2=(V_1d_1^2)/(d_2^2)`

`V_2=(14.71* 8^2)/(4^2)`

`V_2=58.84\ m/s`