Answer:
a) See proof below
b)The result does not violate uniqueness of solutions.
Explanation:
a)
Suppose
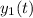 is a solution of
is a solution of

then
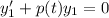
If
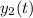 is a solution of
is a solution of
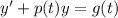
then
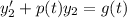
To show that
 is a solution of equation (2) we have to show that
is a solution of equation (2) we have to show that
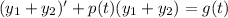
But
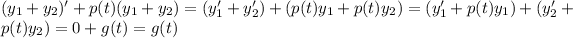
which is what we wanted to show.
b)
The results of the above problem does not violate uniqueness of solutions, because the solution is unique for each specific given initial condition, but there are no initial conditions stated.