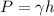Answer:
The pressure is directly proportional to the product of the height of column and the specific weight.
Step-by-step explanation:
This comes from the fact that pressure is defined to be the ratio between the force and the area over which the force is being applied. That's why pressure has units of force over area:
![P=([F])/([A])](https://img.qammunity.org/2020/formulas/engineering/college/r9c9h33wffgb6nb5nwylgis1jlfxueyg5e.png)
now, the sperific weight has dimensions of force over volume:
![\gamma= (F)/([V])](https://img.qammunity.org/2020/formulas/engineering/college/b68z4w9689bq3edylmmbpbm3eyv3qzlktz.png)
and height has dimensions of length
h=L
we know that area is given as the square of length:
![[A]=[L^(2)]](https://img.qammunity.org/2020/formulas/engineering/college/vy74mys4jqkzsm0ohkcj4rmb6p86tp1sys.png)
and volume is given as the cube of length.
![[V]=[L^(3)]](https://img.qammunity.org/2020/formulas/engineering/college/pfod4w67qgc9075v2crdq5kd25p5tqsdjs.png)
so we can use this to relate specific weight and column height.
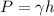
![P=((F)/([L^(3)]))(L)](https://img.qammunity.org/2020/formulas/engineering/college/osfbcxbhnrkd27e2xzoyu19kvygp7zrice.png)
Which leaves us with units of force over area.
![P=([F])/([A])](https://img.qammunity.org/2020/formulas/engineering/college/r9c9h33wffgb6nb5nwylgis1jlfxueyg5e.png)
So the formula for pressure is: