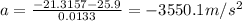Question

A tennis ball with a speed of 25.9 m/s is moving perpendicular to a wall. After striking the wall,

the ball rebounds in the opposite direction with a speed of 21.3157 m/s. If the ball is in contact
with the wall for 0.0133 s, what is the average acceleration of the ball while it is in contact with
the wall? Take "toward the wall” to be the positive direction. Answer in units of m/s 2

Answer 1

`-3550.1 m/s^2`

Step-by-step explanation:

The initial velocity of the ball is

u = +25.9 m/s (towards the wall)

while the final velocity of the ball is

v = -21.3157 m/s (away from the wall)

The time taken for the change in velocity to occur is

t = 0.0133 s

The acceleration can be calculated as the change in velocity divided by the time taken:

`a=(v-u)/(t)`

Substituting the numbers, we find:

`a=(-21.3157-25.9)/(0.0133)=-3550.1 m/s^2`