Question

Radiant heat makes it impossible to stand close to a hot lava flow. Calculate the rate of heat loss by radiation from 1.00 m^2 of 1110°C fresh lava into 36.2°C surroundings, assuming lava's emissivity is 1.

Answer 1

The rate of heat loss by radiation is equal to -207.5kW

Why?

To calculate the heat loss rate (or heat transfer rate) by radiation, from the given situation, we can use the following formula:

`HeatLossRate=E*S*A*((T_(cold))^(4) -(T_(hot))^(4) )`

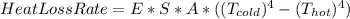
Where,

E, is the emissivity of the body.

A, is the area of the body.

T, are the temperatures.

S, is the Stefan-Boltzmann constant, which is equal to:

`5.67x10^(-8)(W)/(m^(2)*K^(-4) )`

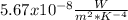
Now, before substitute the given information, we must remember that the given formula works with absolute temperatures (Kelvin), so, we need to convert the given values of temperature from Celsius degrees to Kelvin.

We know that:

`K=Celsius+273.15`

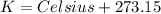
So, converting we have:

`T_(1)=1110\°C+273.15=1383.15K\\\\T_(2)=36.2\°C+273.15=309.35K`

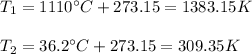
Therefore, substituting the given information and calculating, we have:

`HeatLossRate=E*S*A*((T_(cold))^(4) -(T_(hot))^(4) )`

`HeatLossRate=1*5.67x10^(-8)(W)/(m^(2)*K^(-4) )*1m^(2) *((309.35K)^(4) -(1383.15})^(4) )\\\\HeatLossRate=5.67x10^(-8)(W)/(K^(-4) )*(95697.42K^(4) -3.66x10^(12)K^(4))\\ \\HeatLossRate=5.67x10^(-8)(W)/(K^(-4) )*(-3.66x10^(12) K^(4))=-207522W=-207.5kW`

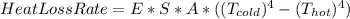
Hence, we have that the rate of heat loss is equal to -207.5kW.