Question

Your favorite professor decided to throw in the air a marker above his head (vertically). If the marker leaves his hand at a height of 1.2 m from the ground with an initial velocity of 20 m/s, when would the marker reach the highest point in the air (1.6 m from the ground)?

Answer 1

`t=4.06s`

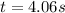
Step-by-step explanation:

From the exercise we know

`y_(o)=1.2m\\v_(o)=20m/s\\y=1.6m\\g=-9.8m/s^2`

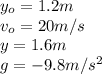
To find how long does it takes the marker to reach the highest point we need to use the equation of position:

`y=y_(o)+v_(oy)t+(1)/(2)gt^(2)`

`1.6m=1.2m+(20m/s)t-(1)/(2)(9.8m/s^2)t^2`

`0=-0.4+20t-4.9t^2`

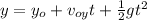
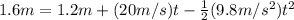
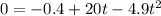
Now, we need to use the quadratic formula:

`t=\frac{-b±\sqrt{b^(2)-4ac } }{2a}`

`a=-4.9\\b=20\\c=-0.4`

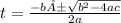
Solving for t

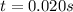`t=0.020s` or
`t=4.06s`

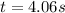
So, the answer is t=4.06s because the other option is almost 0 and doesn't make any sense for the motion of the marker