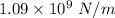Answer:
The spring constant is
Step-by-step explanation:
Given that,
length = 500 mm
Diameter = 2 cm
Young's modulus = 17.4 GPa
We need to calculate the young's modulus
Using formula of young's modulus
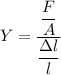 ....(I)
....(I)
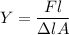
From hook's law
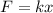
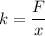
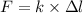 ....(II)
....(II)
Put the value of F in equation
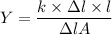
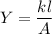
We need to calculate the spring constant
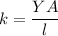 ....(II)
....(II)
We need to calculate the area of cylinder
Using formula of area of cylinder

Put the value into the formula
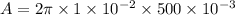
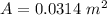
Put the value of A in (II)
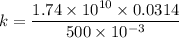
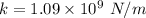
Hence, The spring constant is