Question

A red ball is thrown down with an initial speed of 1.1 m/s from a height of 28 meters above the ground. Then, 0.5 seconds after the red ball is thrown, a blue ball is thrown upward with an initial speed of 24.4 m/s, from a height of 0.9 meters above the ground. The force of gravity due to the earth results in the balls each having a constant downward acceleration of 9.81 m/s^2. How long after the red ball is thrown are the two balls in the air at the same height?

Answer 1

Answer:0.931 s

Step-by-step explanation:

Given

initial speed=1.1 m/s

height(h)=28 m

after 0.5 sec blue ball is thrown upward

Velocity of blue ball is 24.4 m/s

height with which blue ball is launched is 0.9 m

Total distance between two balls is 28-0.9=27.1 m

Let in t time red ball travels a distance of x m

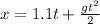`x=1.1t+(gt^2)/(2)` --------1

for blue ball

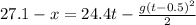`27.1-x=24.4t-(g(t-0.5)^2)/(2)` -----2

Add 1 & 2

we get

`27.1=24.4t+1.1t+(g(2t-0.5)(0.5))/(2)`

`27.1=25.5t+g(4t-1)/(8)`

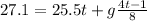
t=0.931 s

after 0.931 sec two ball will be at same height