Question

"The diagram above represents a square garden. If each side of the garden is increased in length by 50%, by what percent is the area of the garden increased?" Whats the explanation for this problem, I cant seem to figure it out?

Answer 1

Explanation:

Let's say that each side of the square is of length
`s`. The area of this square would then be:

`A = s^(2)`

If we increase the length of each side by 50%, then the length becomes
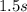`1.5s`, which will result in the area being:

`A = (1.5s)(1.5s)`

`A = 2.25s^(2)`

This means the area has increased by
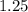`1.25` the original amount, or 125%.