Question

Find the equation of the circle: y-intercepts 4 and –8, contain (–12, –8)

Answer 1

`(x+6)^2 + (y+2)^2 = 72`

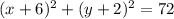
Explanation:

We are given the following information in the question:

y intercept = 4, -8

The circle passes through the point (-12, -8)

Equation of circle:

`(x-h)^2 + (y-k)^2 = r^2`

where r is the radius of circle, (h,k) is the center of circle.

The circle passes through the points (0,4), (0,-8_ and (-12,-8)

Putting these points in the equation of circle we get:

`1) (0-h)^2 + (4-k)^2 = r^2\\h^2 + (4-k)^2 = r^2\\2) (0-h)^2 + (-8-k)^2 = r^2\\h^2 + (-8-k)^2 = r^2\\3) (-12-h)^2 + (-8-k)^2 = r^2\\`

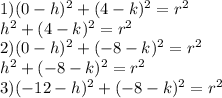
Now, we have three equations in three variables.

Solving the three equations, we obtain:

h = -6, k = -2, r =
`6\sqrt2`

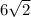
Putting these values in the equation of circle:

`(x-(-6))^2 + (y-(-2)) = (6√(2))^2\\(x+6)^2 + (y+2)^2 = 72`

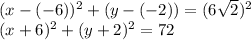
The above equation is the required equation of circle.

Answer 2

`\boxed{(x+6)^2+(y+2)^2=72}`

Explanation:

The center-radius form of the circle equation is given by:

`(x-h)^2+(y-k)^2=r^2`

Here we know that the y-intercepts are:

`y=4 \ and \ y=-8`

So:

`\bullet \ If \ y=4, \ x=0 \\ \\ \\ (0-h)^2+(4-k)^2=r^2 \\ \\ \therefore \mathbf{(I)} \ h^2+16-8k+k^2=r^2 \\ \\ \\ \bullet \ If \ y=-8, \ x=0 \\ \\ \\ (0-h)^2+(-8-k)^2=r^2 \\ \\ \therefore \mathbf{(II)} \ h^2+64+16k+k^2=r^2 \\ \\ \\ \bullet \ If \ x=-12, \ y=-8 \\ \\ \\ (-12-h)^2+(-8-k)^2=r^2 \\ \\ \therefore 144+24h+h^2+64+16k+k^2=r^2 \\ \\ \therefore \mathbf{(III)} \ h^2+208+16k+24h+k^2=r^2`

So we have the following system of equations:

`\left\{ \begin{array}{c}(I)\:h^(2)+16-8k+k^(2)=r^(2)\\(II)\:h^(2)+64+16k+k^(2)=r^(2)\\(III)\:h^(2)+208+16k+24h+k^(2)=r^(2)\end{array}\right.`

`Subtract \ II \ from \ I \\ \\ \\\left\{ \begin{array}{c}h^(2)+16-8k+k^(2)=r^(2)\\-(h^(2)+64+16k+k^(2)=r^(2))\\---------------------\\h^(2)+16-8k+k^(2)-h^(2)-64-16k-k^(2)=r^(2)-r^(2)\end{array}\right.`

`Simplifying: \\ \\ h^(2)+16-8k+k^(2)-h^(2)-64-16k-k^(2)=r^(2)-r^(2)\\ \\ 16-8k-64-16k=0 \\ \\ -24k-48=0 \\ \\ k=-(48)/(24) \\ \\ \therefore \boxed{k=-2}`

From (I):

`h^2+16-8k+k^2=r^2 \\ \\ \\ For \ k=-2 \\ \\ h^2+16-8(-2)+(-2)^2=r^2 \\ \\ \therefore r^2=h^2+36 \\ \\ \\ Substituting \ k \ and \ r^2 \ into \ (III): \\ \\ h^(2)+208+16(-2)+24h+(-2)^(2)=h^2+36 \\ \\ Simplifying: \\ \\ 180+24h=36 \\ \\ 24h=36-180 \\ \\ 24h=-144 \\ \\ h=-(144)/(24) \\ \\ \therefore \boxed{h=-6}`

Finding the radius:

`r^2=(-6)^2+36 \\ \\ r^2=36+36 \\ \\ r^2=72 \\ \\ \therefore \boxed{r=6√(2)}`

Finally, the equation of the circle is:

`(x-(-6))^2+(y-(-2))^2=72 \\ \\ \boxed{(x+6)^2+(y+2)^2=72}`