Question

in a geometric progression ,the sum of 3rd and 4th term is 108. if the sum of the 4th and 5th term is 324, determine the: common ratio, first term and the 12th term.​

Answer 1

see explanation

Explanation:

The terms of a geometric progression are

a, ar, ar², ar³, ...... , a
`r^(n-1)`

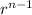
Thus the sum of the third and fourth is

ar² + ar³ = 108 → (1)

The sum of the fourth and fifth is

ar³ + a
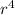`r^(4)` = 324 → (2)

Factorise both equations

ar²(1 + r) = 108 → (3)

ar³(1 + r) = 324 → (4)

Divide (4) by (3)

`(ar^3(1+r))/(ar^2(1+r))` =
`(324)/(108)`

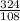
Cancelling (1 + r) , a and r, gives

r = 3

Substitute r = 3 into (3) and solve for a

9a(4) = 108

36a = 108 ( divide both sides by 36 )

a = 3

The n th term is

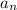`a_(n)` = a
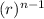`(r)^(n-1)` with a = 3 and r = 3, hence

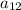`a_(12)` = 3
`(3)^(11)` = 531441