Question

Write the equation, in general form, of the line that passes through the given points.

y - x + 1 = 0
x - y + 1 = 0
-x - y + 1 = 0
x + y + 1 = 0

Answer 1

Answer : The correct option is, (x-y+1=0)

Step-by-step explanation :

The general form for the formation of a linear equation is:

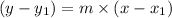`(y-y_1)=m* (x-x_1)` .............(1)

where,

x and y are the coordinates of x-axis and y-axis respectively.

m is slope of line.

First we have to calculate the slope of line.

Formula used :

`m=((y_2-y_1))/((x_2-x_1))`

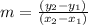
Here,

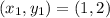`(x_1,y_1)=(1,2)` and
`(x_2,y_2)=(4,5)`

`m=((5-2))/((4-1))`

`m=(3)/(3)`

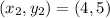
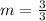
m = 1

Now put the value of slope in equation 1, we get the linear equation.

`(y-y_1)=m* (x-x_1)`

`(y-2)=1* (x-1)`

`(y-2)=(x-1)`

`y-2=x-1`

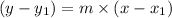
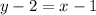
Now rearranging the terms, we get:

`y-x-1=0`

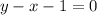
or,

`x-y+1=0`

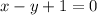
From the given options we conclude that the option (x-y+1=0) is an equation of the given line in standard form.

Hence, the correct option is, (x-y+1=0)

Answer 2

x + y + 1 = 0

Explanation:

Equation of line in general form ⇒ mx + y + c = 0

Step 1: Find slope (m)

Formula for slope = y2-y1/x2-x1

(4,5) (1,2)

m = 2-5/1-4

m = -3/-3

m = 1

Step 2: Find y-intercept where x = 0

⇒ when x = 0, y = -1

mx + y + c = 0

1(0) - 1 + c = 0

c = 1

Step 3: Write in general form

⇒ m = 1

⇒ c = 1

y=mx + c

mx + c - y = 0

mx - y + c = 0

1(x) - y + 1 = 0

x - y + 1 = 0

Therefore, option c is correct where the general equation is x -y + 1 = 0.