Question

CS2(g) + O2(g) CO2(g) + SO2(g)

Consider the unbalanced equation above. A 0.150 g sample of CS2 is reacted with 285 mL of O2 at STP. What is the total volume of the final mixture of gases at STP?

Answer 1

Step-by-step explanation:

Given parameters:

Mass of CS₂ = 0.15g

Volume of O₂ = 285mL

Condition of reaction = at standard temperature and pressure.

Solution:

Balanced reaction equation is shown below:

CS₂ + 3O₂ → CO₂ + 2SO₂

For gases at standard temperature and pressure(STP);

number of moles =
`(volume occupied)/(22.4)`

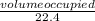
To find the final mixture of the gases at STP, we must identify the limiting reactant here first. The limiting reactant determines the extent of the reaction since it is the one used up.

Number of moles of CS₂ =
`(mass)/(molar mass)`

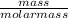
molar mass of CS₂ = 12 + (2 x 32) = 76g/mol

Number of moles of CS₂ =
`(0.15)/(76)` = 0.00197mole

Number of moles of O₂ =
`(volume occupied)/(22.4)`

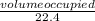
converting mL to dm³;

1000mL = 1dm³

285mL = 0.285dm³

Number of moles of O₂ =
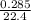`(0.285)/(22.4)` = 0.01272mole

From the reaction equation:

1 mole of CS₂ reacted with 3 moles of O₂;

0.00197 mole of CS₂ will require 3 x 0.00197; 0.00591mole to react with;

We see that we have more than enough O₂. CS₂ is the limiting reagent.

Number of moles of excess O₂ = 0.01272 - 0.00591 = 0.00681moles

Now, we use the information of the limiting reagent to determine the product,

1 mole of CS₂ will produce 1 mole of CO₂;

0.00197 mole of CS₂ will produce 0.00197 mole of CO₂

1 mole of CS₂ will produce 2 mole of SO₂

0.00197mole of CS₂ will produce 2 x 0.00197; 0.00394mole of SO₂

The moles of the final mixture will = number of moles of excess O₂ + number of moles of CO₂ + number of moles of SO₂ = 0.00681+0.00197+0.00394

= 0.01272moles

Volume of the final mixture = number of moles x 22.4 = 0.01272 x 22.4

Volume of final mixture = 0.2849dm³ or 284.9mL