Answer:
(a) 52.724 m/s
(b) Total displacement, d = 551.25 m
Solution:
As per the question:
Initial acceleration of the speed boat, a = - 2.01
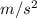
Time duration, t = 7.00 s
Additional time, t' =6.00 s
Acceleration for additional time, a' = 0.518
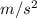
The followed up acceleration, a'' = 1.49
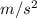
Time duration, t'' = 8.00 s
(a) Now, to calculate the velocity of the boat at timer, t = 21.0 s, we have:
After the initial 7.00 s, the velocity of the boat, from eqn-1 of motion:
v = u + at
v = 0 - 2.1(7.00) = - 14.7 m/s
After t + t' = 13 s:
v' = v + at
v' = 14.7 + 0.518(13) = 21.434 m/s
Now, velocity of the boat after t = 21 s:
v'' = v' + a''t
v'' = 21.434 + 1.49(21) = 52.724 m/s
(b) Now, the total displacement, d:
For the first case:
d = ut +
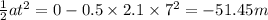
For the second case:
d = v't' = 21.434(6) = 128.6 m
For the third case:
d = ut +
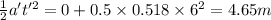
For the fourth case:
d = v''t'' = 52.724(8) = 421.79 m
For the last case:
d = ut +
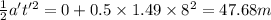
Total displacement, d = -51.45 + 128.6 + 4.65 + 421.79 + 47.68 = 551.25 m