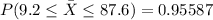Answer:
a.
 is distributed
is distributed
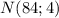
b.

c.

d.
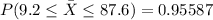
Explanation:
a.
The central limit theorem states that, for large n, the sampling distribution of the sample mean is approximately normal with mean
 and variance
and variance
 , then, the sample mean is distributed as a normal random variable with means
, then, the sample mean is distributed as a normal random variable with means
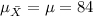 and variance
and variance
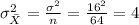 .
.
b.

c.

d.