Answer:
Christina is 10 years old now
Explanation:
- Right now Christina is twice as old as mark
- Assume that mark now is x years old
∵ Mark is x years old
∵ Christina is twice as old as mark
∴ Christina is 2 x years old
- In five years, she will be 50% older than him
∵ Mark is x years old now
∴ In five years mark will be x + 5 years old
∵ Christina is 2 x years old now
∴ In five years Christina will be 2 x + 5 → (1)
∵ In five years Christina will be 50% older than him
- Assume that he will be 100% year and she is older by 50%, then she
will be 150% ⇒ (100% + 50%)
∵ 150% =
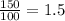
∴ In five years Christina will be 1.5(x + 5) → (2)
- Equate (1) and (2)
∴ 2 x + 5 = 1.5(x + 5)
- Simplify the right hand side
∴ 2 x + 5 = 1.5 x + 7.5
- Subtract 1.5 x from both sides
∴ 0.5 x + 5 = 7.5
- Subtract 5 from both sides
∴ 0.5 x = 2.5
- Divide both sides by 0.5
∴ x = 5
∵ x represents the age of Mark now
∴ Mark now is 5 years old
∵ Christina is twice as old as Mark now
∴ Christina is 10 years old
* Christina is 10 years old now