Question

Three forces act on a moving object. One force has a magnitude of 83.7 N and is directed due north. Another has a magnitude of 59.5 N and is directed due west. What must be (a) the magnitude and (b) the direction of the third force, such that the object continues to move with a constant velocity? Express your answer as a positive angle south of east.

Answer 1

• 
  `|\vec{F}_3| = 102.92 \ N`

• 
  `\theta = 57 \° 24 ' 48''`

Step-by-step explanation:

For an object to move with constant velocity, the acceleration of the object must be zero:

`\vec{a} = \vec{0}`.

As the net force equals acceleration multiplied by mass , this must mean:

`\vec{F}_(net) = m \vec{a} = m * \vec{0} = \vec{0}`.

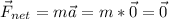So, the sum of the three forces must be zero:

`\vec{F}_1 + \vec{F}_2 + \vec{F}_3 = \vec{0}`,

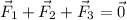this implies:

`\vec{F}_3  = - \vec{F}_1 - \vec{F}_2`.

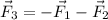To obtain this sum, its easier to work in Cartesian representation.

First we need to define an Frame of reference. Lets put the x axis unit vector
`\hat{i}` pointing east, with the y axis unit vector
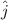`\hat{j}` pointing south, so the positive angle is south of east. For this, we got for the first force:

`\vec{F}_1 = 83.7 \ N \ (-\hat{j})`,

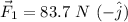as is pointing north, and for the second force:

`\vec{F}_2 = 59.9 \ N \ (-\hat{i})`,

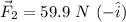as is pointing west.

Now, our third force will be:

`\vec{F}_3  = - 83.7 \ N \ (-\hat{j}) - 59.9 \ N \ (-\hat{i})`

`\vec{F}_3  =  83.7 \ N \ \hat{j}  + 59.9 \ N \ \hat{i}`

`\vec{F}_3  =  (59.9 \ N , 83.7 \ N )`

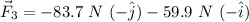
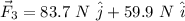
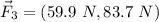
But, we need the magnitude and the direction.

To find the magnitude, we can use the Pythagorean theorem.

`|\vec{R}| = √(R_x^2 + R_y^2)`

`|\vec{F}_3| = √((59.9 \ N)^2 + (83.7 \ N)^2)`

`|\vec{F}_3| = 102.92 \ N`

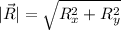
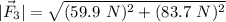
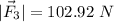
this is the magnitude.

To find the direction, we can use:

`\theta = arctan((F_(3_y))/(F_(3_x)))`

`\theta = arctan((83.7 \ N )/( 59.9 \ N ))`

`\theta = 57 \° 24 ' 48''`

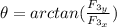
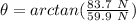
and this is the angle south of east.