Answer:
The confidence interval is 6.6<μ<6.8.
Explanation:
We have:
Number of observations = 601
Mean = 6.7
Standard deviation σ = 1.5
The z-score for a 95% confidence interval is 1.96.
The limits of the confidence interval can be calculated as
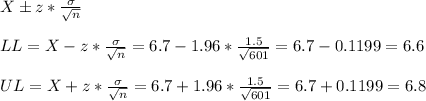
The confidence interval is 6.6<μ<6.8.