Question

Seattle Radiology Group plans to invest in a new CT scanner. The group estimates $1,500 net revenue per scan. Preliminary market assessments indicate that demand will be less than 5,000 scans per year. The group has the choice between two different types of scanner that can fill its imaging needs. Each scanner has a capacity of 5,000 scans per year but involves a different mix of labor and capital. Scanner A would result in total fixed costs of $1,000,000 per year and would yield a profit of $500,000 if the volume is 5,000 scans. Scanner B would result in total fixed costs of $800,000 per year and would yield a profit of $450,000 if the volume is 5,000 scans. At what number of scans are the scanners equally profitable?

Answer 1

Answer: 4,000 scans

Step-by-step explanation:

Scanner A:

Total revenue = Volume × Revenue per scan

= 5,000 x 1,500

= $7,500,000

Profit = Total revenue - Total Variable cost - Total fixed cost

Total Variable cost = Total revenue - Total fixed cost - Profit

= $7,500,000 - 1,000,000 - 500,000

= $6,000,000

`variable\ cost\ per\ scan=(6,000,000)/(5,000)`

= $1,200

Scanner B:

Total revenue = Volume × Revenue per scan

= 5,000 x 1,500

= $7,500,000

Profit = Total revenue - Total Variable cost - Total fixed cost

Total Variable cost = Total revenue - Total fixed cost - Profit

= $7,500,000 - 800,000 - 450,000

= $6,250,000

`variable\ cost\ per\ scan=(6,250,000)/(5,000)`

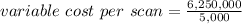
= $1,250

Let X be the number of scans where total profits of the two scanners are same.

Profit from scanner A = Total revenue - Total Variable cost - Total fixed cost

= 1,500 × X - 1,200 × X - 1,000,000

= 1,500X - 1,200X - 1,000,000

= 300X - 1,000,000

Profit from scanner B = Total revenue - Total Variable cost - Total fixed cost

= 1,500 × X - 1,250 × X - 800,000

= 1,500X - 1,250X - 800,000

= 250X - 800,000

Therefore,

300X - 1,000,0000 = 250X - 800,000

50X = 200,000

X = 4,000

Hence when 4,000 scans are done, total profits of the two scanners would be same.