Answer:
The correct answer is D.
Explanation:
In order to obtain the correct answer we need to recall the definition of function (or map) from one set to another. So, we say that a function (or map) is a way to associate a unique object to every element of a set. In a more mathematical formulation we say that
 is a function if, for every element
is a function if, for every element
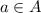 there exists a unique element
there exists a unique element
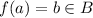 .
.
We need to recall the definition of linear transformation too. So, we say that a map
 is a linear transformation such that
is a linear transformation such that
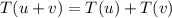 , for all
, for all
 ,
,
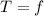 .
.
Also, a linear transformation must satisfies the conditions stated in its definition.
Just notice that these are the explanation that comes with the option D.