Answer:
The absolute maximum of
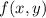 in the triangle plate is 20, and the absolute minimum is 0.
in the triangle plate is 20, and the absolute minimum is 0.
Explanation:
We have the function
 and we want to the the absolute maximum and minimum in the bounded region determined by the lines
and we want to the the absolute maximum and minimum in the bounded region determined by the lines
 ,
,
 and
and
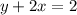 , and we will call it
, and we will call it
 .
.
Usually, in this kind of problem, the first step is to assure the existence of the absolute maximum and minimum. The general procedure is to show that the function is continuous and the region is closed and bounded.
It is no difficult to see that the function
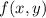 is continuous in the whole plane, so it is in the given region. Moreover, the region is closed and bounded. So, we can assure the existence of the absolute minimum and maximum of
is continuous in the whole plane, so it is in the given region. Moreover, the region is closed and bounded. So, we can assure the existence of the absolute minimum and maximum of
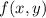 in the triangle plate.
in the triangle plate.
The second step is to find the critical point(s) of the function in the interior of
 . In order to do that we must calculate the partial derivatives:
. In order to do that we must calculate the partial derivatives:
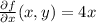
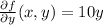
Recall that the critical points are those that makes zero both partial derivatives. So, we need to solve the system of equations

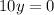
that has as only solution the point
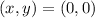 . Now, the point (0,0) is not in the interior of
. Now, the point (0,0) is not in the interior of
 but in the boundary.
but in the boundary.
As the function has no critical points inside the region
 , we must look for the extremal values of
, we must look for the extremal values of
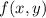 in the boundary of
in the boundary of
 .
.
In order to make the following explanation simpler there is a figure attached, where the triangle plate
 has been drawn.
has been drawn.
Now, we need to give ‘‘parametric’’ expressions for the boundary of the set
 . Notice that:
. Notice that:
- The segment AC has the form
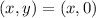 with
with
![x\in[0,1]](https://img.qammunity.org/2020/formulas/mathematics/college/njp3y9dszw8kotirkf8gsiyuttfnh6vb6w.png) .
. - The segment AB has the form
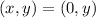 with
with
![y\in[0,2]](https://img.qammunity.org/2020/formulas/mathematics/college/o53corl8umhdfssjemn9ctn9bav6h6lpa6.png) .
. - The segment BC has the form
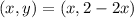 with
with
![x\in[0,1]](https://img.qammunity.org/2020/formulas/mathematics/college/njp3y9dszw8kotirkf8gsiyuttfnh6vb6w.png)
The last representation was deduced from the equation of the line, because
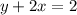 is equivalent to
is equivalent to
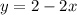 .
.
Now, let us analyze the behavior of
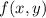 in each of the sides of the triangle. The procedure will be to find the maximal and minimum of
in each of the sides of the triangle. The procedure will be to find the maximal and minimum of
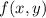 in each side of the triangle, and finally we will compare them and take the minimal value as the absolute minimum and the maximal as the absolute maximum.
in each side of the triangle, and finally we will compare them and take the minimal value as the absolute minimum and the maximal as the absolute maximum.
The segment AC. In this case we have
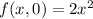 with
with
![x\in[0,1]](https://img.qammunity.org/2020/formulas/mathematics/college/njp3y9dszw8kotirkf8gsiyuttfnh6vb6w.png) . So, we have reduced our two variable function to one variable function, which has a simpler behavior. Here, we can affirm that the maximal value of
. So, we have reduced our two variable function to one variable function, which has a simpler behavior. Here, we can affirm that the maximal value of
 in AC is obtained when
in AC is obtained when
 and is
and is
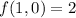 , and the minimal is obtained when
, and the minimal is obtained when
 and is
and is
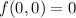 .
.
The segment AB. In this case we have
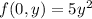 with
with
![y\in[0,2]](https://img.qammunity.org/2020/formulas/mathematics/college/o53corl8umhdfssjemn9ctn9bav6h6lpa6.png) . So, again, we have reduced our two variable function to one variable function, which has a simpler behavior. Here, we can affirm that the maximal value of
. So, again, we have reduced our two variable function to one variable function, which has a simpler behavior. Here, we can affirm that the maximal value of
 in AB is obtained when
in AB is obtained when
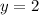 and is
and is
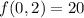 , and the minimal is obtained when
, and the minimal is obtained when
 and is
and is
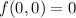 .
.
The segment BC. In this case we have
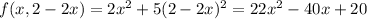 with
with
![x\in[0,1]](https://img.qammunity.org/2020/formulas/mathematics/college/njp3y9dszw8kotirkf8gsiyuttfnh6vb6w.png) .
.
So, we have reduced our two variable function to one variable function. In order to obtain the extremal values of
 in BC, we must find the extremal values of
in BC, we must find the extremal values of
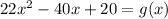 in the interval [0,1]. Though not so easy as the previous two, this problem is easier that the original.
in the interval [0,1]. Though not so easy as the previous two, this problem is easier that the original.
So, we calculate the derivative of the function
 :
:
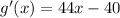 , which has only one zero in the interval [0,1] and it is 40/44. To obtain the minimum and maximum of
, which has only one zero in the interval [0,1] and it is 40/44. To obtain the minimum and maximum of
 in [0,1] we only need to evaluate
in [0,1] we only need to evaluate
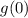 ,
,
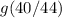 and
and
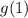 . Then,
. Then,
So, we can affirm that the maximal value of
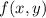 in BC is obtained when
in BC is obtained when
 and is
and is
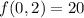 , and the minimal is obtained when
, and the minimal is obtained when
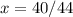 and is
and is
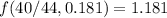 .
.
Conclusion: The extremal values of
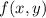 obtained in the boundary of the triangle are:
obtained in the boundary of the triangle are:
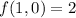 and
and
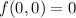 .
.
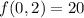 and
and
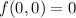 .
.
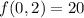 and
and
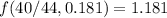 .
.
So, of all those values the minimum is
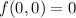 and the maximum is
and the maximum is
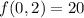 . This means that
. This means that

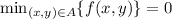 .
.