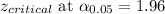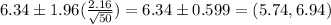Answer:
Confidence interval: (5.74,6.94)
Explanation:
We are given the following data set:
6, 4, 6, 8, 7, 7, 6, 3, 3, 8, 10, 4, 8, 7, 8, 7, 5, 9, 5, 8, 4, 3, 8, 5, 5, 4, 4, 4, 8, 4, 5, 6, 2, 5, 9, 9, 8, 4, 8, 9, 9, 5, 9, 7, 8, 3, 10, 8, 9, 6
Formula:
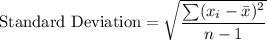
where
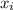 are data points,
are data points,
 is the mean and n is the number of observations.
is the mean and n is the number of observations.
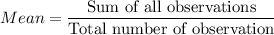
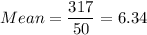
Sum of squares of differences = 0.1156+5.4756+0.1156+2.7556+0.4356+0.4356+0.1156+11.1556+11.1556+2.7556+13.3956+5.4756+2.7556+0.4356+2.7556+0.4356+1.7956+7.0756+1.7956+2.7556+5.4756+11.1556+2.7556+1.7956+1.7956+5.4756+5.4756+5.4756+2.7556+5.4756+1.7956+0.1156+18.8356+1.7956+7.0756+7.0756+2.7556+5.4756+2.7556+7.0756+7.0756+1.7956+7.0756+0.4356+2.7556+11.1556+13.3956+2.7556+7.0756+0.1156 = 229.22

Confidence interval:
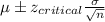
Putting the values, we get,