Question

The skateboarder in the drawing starts down the left side of the ramp with an initial speed of 7.03 m/s. Neglect nonconservative forces, such as friction and air resistance, and find the height h of the highest point reached by the skateboarder on the right side of the ramp. (g = 9.80 m/s2)

Answer 1

The height of the highest point reached by the skateboarder on the right side of the ramp is
`h=2.52m`

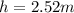
Step-by-step explanation:

In this problem, we use the Energy Conservation Principle, as there are not nonconservative forces, the energy is constant and we will call it E. Then, we choose two different moments that will be advantageous for our analysis.

The first moment is the moment where the skateboarder is situated at the height 0 meters (meaning that the potential energy is zero), i.e, the energy is totally kinetic. We will call it K.

The second moment is the moment where the skateboarder is situated at the highest point reached, this means that all energy will be potential, because for an instant, the velocity of the skateboarder is zero (and in the following instant he will go back the way he came due to the pull of gravity). We will call it V. This is the point with the height that we want to calculate.

Therefore, we can equalize both energies, as they are constant and equal to E. We write

`E=K=V=(1)/(2)mv^2 =mgh\Leftrightarrow (1)/(2)v^2=gh\Leftrightarrow h=(v^2)/(2g)`

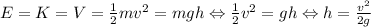
Now, we can replace the given data, to obtain

`h=(7.03^2)/(2*9.80)m=2.52m`

which is the outcome.

Answer 2

h=5.04m

Step-by-step explanation:

At the bigining he has only kinetic energy because he is at the lowest point. When he is at the highest point, he is no longer moving because he will start moving downwards: all his kinetic energy transformed into potential gravitational energy:

`E_k=E_p`

`(mv^2)/(2)=mgh\\ h= (v^2)/(g)`

h=5.04m