Question

PI-3.

FIELD TRIP
In order to take a field trip to the science museum. Ms. Speedi's students sold
scented pencils for $1. In the classroom. Rowena tried to put the $1 bills into a
equal piles and found one left over at the end. When Polly tried putting the bin
in 3 equal piles, she also ended up with one extra bill. Frustrated, they tried 4
5, and 6 equal piles and each time had $1 left over. Finally Rowena put all the
bills evenly into 7 equal piles, and none were left over.
Based on this information, Ms. Speedi is sure there is enough money for the
fieldtrip!
a. What is the least amount of money they could have raised? Show how
know.
b.
It turns out that they raised more than $500. Knowing this, what is the least
amount of money they could have raised?​

Answer 1

A:301 and b: 721

Answer 2

A. $301

B. $721

Explanation:

Let $x be the amount of money they raised.

Rowena tried to put the $1 bills into two equal piles and found one left over at the end, then

`x=2q_1+1`

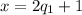
Polly tried to put the $1 bills into three equal piles and found one left over at the end, then

`x=3q_2+1`

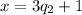
Frustrated, they tried 4, 5, and 6 equal piles and each time had $1 left over, then

`x=4q_3+1\\ \\x=5q_4+1\\ \\x=6q_5+1`

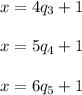
Finally Rowena put all the bills evenly into 7 equal piles, and none were left over, then

`x=7q_6`

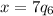
This means
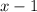`x-1` is divisible by 2, 3, 4, 5 and 6 without remainder, so

`x-1=2\cdot 3\cdot 2\cdot 5n=60n`

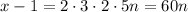
Hence,

`x=60n+1, \ n\in N`

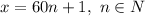
The smallest amount of money they could have raised is $301, because

`x=60\cdot 5+1=301` is divisible by 7.

Now, the number
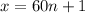`x=60n+1` should be divisible by 7 and must be greater than 500.

So,

`60n+1>500\\ \\60n>499\\ \\n>8`

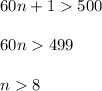
When n = 9,

`x=60\cdot 9+1=541` is not divisible by 7.

When n = 10,

`x=60\cdot 10+1=601` is not divisible by 7.

When n = 11,

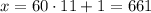`x=60\cdot 11+1=661` is not divisible by 7.

When n = 12,

`x=60\cdot 12+1=721` is divisible by 7.

B. The least amount of money they could have raised is $721