Question

The measure of an exterior angle of a regular polygon is given below. Find the measure of an interior angle. Then find the number of sides. 20

Answer 1

Internal angle =
`160^\circ`

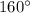
Number of sides = 18

Explanation:

Given that:

We have a regular polygon with number of sides unknown.

Measure of an exterior angle of the polygon =
`20^\circ`

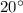
To find:

Measure of an interior angle and the number of sides of the polygon?

Solution:

Let us have a look at the relation of sum of external angles.

Sum of one internal angle and its corresponding external angle =
`180^\circ`

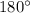
Internal angle + external angle =
`180^\circ`

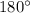
Internal angle =
`180^\circ` -
`20^\circ` =
`160^\circ`

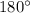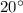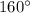
Sum of all the external of a regular polygon =
`360^\circ`

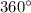
All the external angles will be equal because it is a regular polygon.

Let the number of sides =
`n`

Therefore,

`n * 20^\circ = 360^\circ\\\Rightarrow n = 18`

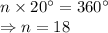
Therefore, the answers are:

Internal angle =
`160^\circ`

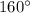
Number of sides = 18