Answer:
The initial value is 29.5
Explanation:
we know that
The linear equation in slope intercept form is equal to

where
m is the slope
b is the y-intercept ( also called initial value)
we have the points
(3,42.25) and (5,50.75)
Find the slope m
The formula to calculate the slope between two points is equal to
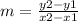
substitute the values

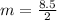
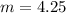
substitute in the equation
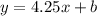
Find the value of b
with the point (3,42.25) ( or the other given point)
substitute the value of x and the value of y in the equation and solve for b
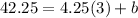
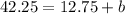
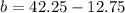
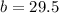
substitute
The linear equation is
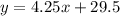
The initial value is the y-intercept (value of y when the value of x is equal to zero)
For x=0

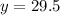
Therefore
The initial value is 29.5