Question

A coffee mixture has beans that sell for $0.20 a pound and beans that sell for $0.68. If 120 pounds of beans create a mixture worth $0.54 a pound, how much of each bean is used? Model the scenario then solve it. Then, in two or more sentences explain whether your solution is or is not reasonable.

Plz give me a step by step answer i need to figure this out.

Answer 1

Explanation:

$ 0.68 type →85lb

$ 0.20 type →35lb

Answer 2

Hey!

-----------------------------------------------

Let $0.68 per pound beans be (b)

Let $0.20 per pound beans be (p)

-----------------------------------------------

~Total amount of pounds:

b + p = 120

~Total cost

b + p = 120 × 0.54

b + p = 64.8

~Expression

0.68b + 0.20p = 64.8

~Multiply 10 to both sides

0.68b * 10 + 0.20p * 10 = 64.8 * 10

~Simplify

6.8b + 2p = 648

~Divide 2 to both sides

6.8b/2 + 2p/2 = 648/2

~Simplify

3.4b + p = 324

~Subtract

3.4b + p = 324

- b + p = 120

~Simplify

2.4b = 204

~Divide 2.4 to both sides

2.4b/2.4 = 204/2.4

~Value of b

b = 85

~New expression

85 + p = 120

~Subtract 85 to both sides

85 + p - 85 = 120 - 85

~Value of p

p = 35

-----------------------------------------------

`\large\boxed{Of~the~0.68~dollars~per~pound~there~are~85~pounds!}\\\large\boxed{Of~the~0.20~dollars~per~pound~there~are~35~pounds!}`

-----------------------------------------------

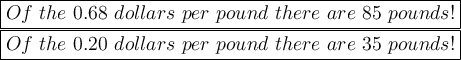
My answer is reasonable because 85 + 35 equals 120 pounds.

~If we check

85 * 0.68 = 57.8

35 * 0.2 = 7

57.8 + 7 = 64.8

We get 64.8 which we can say it is correct because if we go back into our work we can see that we used the expression b + p = 64.8 which in this case by checking our work our answer is reasonable.

-----------------------------------------------

Hope This Helped! Good Luck!