Answer:
The value of x in log x + log 3 = log 18 is 6.
Solution:
From question, given that log x + log 3 = log 18 ---- eqn 1
Let us first simplify left hand side in above equation,
We know that log m + log n = log (mn) ----- eqn 2
Adding log m and log n results in the logarithm of the product of m and n (log mn)
By using eqn 2, log x + log 3 becomes log 3x.
log x + log 3 = log 3x ---- eqn 3
By substituting eqn 3 in eqn 1, we get
log 3x = log 18
Since we have log on both sides, we can cancel log and the above equation becomes,
3x = 18
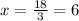
Thus the value of x in log x + log3 = log18 is 6