Step-by-step explanation:
The given data is as follows.
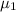 = 0.1 Pa.s,
= 0.1 Pa.s,
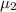 = 0.2 Pa.s
= 0.2 Pa.s
 =
=
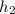 = 1 mm =
= 1 mm =
 (as 1 m = 1000 mm)
(as 1 m = 1000 mm)
As the velocity gradients are linear so, the shear stress will be the same throughout.
 = velocity at the interface
= velocity at the interface
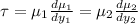
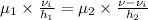
or,
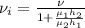
Now, putting the given values into the above formula as follows.
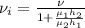
=
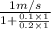
= 0.667 m/s
Hence, force required will be F =

or, F =
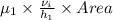
=
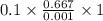
= 66.67 N
Thus, we can conclude that the fluid velocity is 0.667 m/s and force necessary to make the upper plate move at a speed U = 1 m/s is 66.67 N.