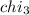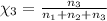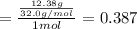Question

A liquid mixture contains water (H2O, MW = 18.0), ethanol (C2H5OH, MW = 46.0) and methanol (CH3OH, MW = 32.0). Using two different analytical techniques to analyze the mixture, it was determined that the water mole fraction was 0.250 while the water mass fraction was 0.134. Determine the mole fraction ethanol (C2H5OH) and the mole fraction methanol (CH3OH) in the solution. Report the values to the correct number of significant figures.

Answer 1

Mole fraction of ethanol is 0.363.

Mole fraction of methanol is 0.387.

Step-by-step explanation:

Mole fraction of water =
`\chi_1=0.250`

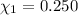
Mole fraction of ethanol =
`\chi_2`

Mole fraction of methanol =
`\chi_3`

`\chi_1+\chi_2+\chi_3=1`

`\chi_2+\chi_3=1-\chi_1=0.750`

`\chi_2+\chi_3=0.750`

`\chi_1=(n_1)/(n_1+n_2+n_3)`

`\chi_2=(n_2)/(n_1+n_2+n_3)`

`\chi_3=(n_3)/(n_1+n_2+n_3)`

`n_1+n_2+n_3=1`

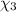
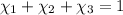
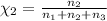
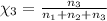
Moles of water =
`n_1=0.250 mol`

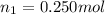
Moles of ethanol=
`n_2`

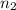
Moles of methanol=
`n_3`

`n_2+n_3=0.750 mol`

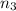
Mass of the mixture = M

Mass of water,
`m_1`

`=n_1* 18.0 g/mol=0.250 mol* 18.0 g/mol=4.5 g`

Fraction of water by mass = 0.134

`(n_1* 18.0 g/mol)/(M)=0.134`

`M=(0.250 mol* 18.0 g/mol)/(0.134)=33.58 g`

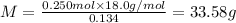
Mass of ethanol =
`m_2`

Mass of methanol =
`m_3`

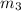
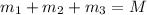
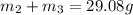`m_1+m_2+m_3=M`

`4.5 g+m_2+m_3=33.58 g`

`m_2+m_3=29.08 g`..[1]

`(m_2)/(46.0 g/mol)+(m_3)/(32.0 g/mol)=0.750 mol`

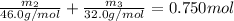
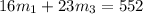`16m_1+23m_3=552`..[2]

On solving [1] and [2]:

`m_2 = 16.70, m_3= 12.38 g`

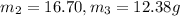
Mole fraction of ethanol =
`chi_2`

`\chi_2=(n_2)/(n_1+n_2+n_3)`

`=((16.70 g)/(46.0 g/mol))/(1 mol)= 0.363`

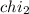
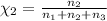
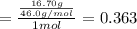
Mole fraction of methanol =
`chi_3`

`\chi_3=(n_3)/(n_1+n_2+n_3)`

`=((12.38 g)/(32.0 g/mol))/(1 mol)= 0.387`