Question

A water treatment plant has 4 settling tanks that operate in parallel (the flow gets split into 4 equal flow streams), and each tank has a volume of 600 m3 . If the total flow to the plant is 12 MGD, what is the retention time in each of the settling tanks? b) If, instead, the tanks operated in series (the entire flow goes first through one tank, then the second, and so on), what would be the retention time in each tank?

Answer 1

a) When the 4 tanks operate in parallel the retention time is 1.26 hours.

b) If the tanks are in series, the retention time would be 0.31 hours

Step-by-step explanation:

The plant has 4 tanks, each tank has a volume V = 600
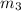`m_3`. The total flow to the plant is Ft = 12 MGD (Millions of gallons per day)

When we use the tanks in parallel, it means that the total flow will be divided in the total number of tanks. F1 will be the flow of each tank.

Firstly, we should convert the MGD to
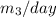`m_3 /day`. In that sense, we can calculate the retention time using the tank volume in
`m_3`.

`Ft =12 (MG)/(day)  ((1*10^6 gall)/(1MG) ) ((3.78 L)/(1 gall) ) ((1 dm^3)/(1L) ) ((1 m^3)/(10^3 dm^3) ) = 45360 (m^3)/(day)`

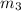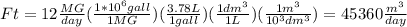
After that, we should divide the total flow by four, because we have four tanks.

`F1 = Ft/4 =(45360 (m^3)/(d) )/4 = 11 340 (m^3)/(d)`

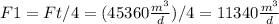
To calculate the retention time we divide the total volume V by the flow of each tank F1.

`t1 =(V1)/(F1) = (600 m^3)/(11340  (m^3)/(d) )  = 0.0529 day\\t1 = 0.0529 d ((24 h)/(1d) ) = 1.26 h`

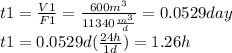
After converting t1 to hours we found that the retention time when the four reactors are in parallel is 1.26 hours.

b)

If the four reactors were working in series, the entire flow goes first through one tank, then the second and so on. It means the total flow will be the flow of each tank.

In that order of ideas, the flow for reactors in series will be F2, and will have the same value of F0.

F2 = F0

To calculate the retention time t2 we divide the total volume V by the flow of each tank F2.

`t2 =(V1)/(F2) = (600 m^3)/(45360  (m^3)/(d) )  = 0.01 day\\t2 = 0.01  d ((24 h)/(1d) ) = 0.31 h`

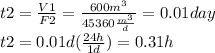
After converting t2 to hours we found that the retention time when the four reactors are in series is 0.31 hours.