Question

The intersection angle of a 3 degree curve is 45.2 degrees. What is the length of the curve? of Select one: O a. 455 m O b.573 m C. 452 m O d. 25.9 km

Answer 1

Step-by-step explanation:

Relation between length of a curve and angle is as follows.

l =
`R * \Theta`

where, R = radius of curve

`\Theta` = angle in radians

Also, l =
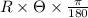`R * \Theta * (\pi)/(180)` .......... (1)

If curve has a degree of curvature
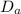`D_(a)` for standard length s, then

R =
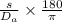`(s)/(D_(a)) * (180)/(\pi)` ........... (2)

Now, substitute the value of R from equation (2) into equation (1) as follows.

l =
`(s * \Theta)/(D_(a))`

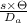
If s = 30 m, then calculate the value of l as follows.

l =
`(s * \Theta)/(D_(a))`

=
`30 * (45.2)/(3)`

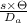
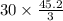
= 452 m

thus, we can conclude that the length of the curve is 452 m.