Question

You notice that the water in your friend's swimming pool is cloudy and that the pool walls are discolored at the water line. A quick analysis reveals that the pH of the water is 8.00 when it should be 7.20. The pool is 9.00 m wide, 15.0 m long, and has an average depth of 2.50m. What is the minimum (in the absence of any buffering capacity) volume (mL) of 12.0 wt% H2SO4 (SG=1.080) that should be added to return the pool to the desired pH? in mL

Answer 1

6,78 mL of 12,0 wt% H₂SO₄

Step-by-step explanation:

The equilibrium in water is:

H₂O (l) ⇄ H⁺ (aq) + OH⁻ (aq)

The initial concentration of [H⁺] is 10⁻⁸ M and final desired concentration is [H⁺] =
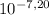`10^(-7,20)`, thus,

Thus, you need to add:

[H⁺] =
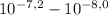`10^(-7,2) -10^(-8,0)` = 5,31x10⁻⁸ M

The total volume of the pool is:

9,00 m × 15,0 m ×2,50 m = 337,5 m³ ≡ 337500 L

Thus, moles of H⁺ you need to add are:

5,31x10⁻⁸ M × 337500 L = 1,792x10⁻² moles of H⁺

These moles comes from

H₂SO₄ → 2H⁺ +SO₄²⁻

Thus:

1,792x10⁻² moles of H⁺ ×
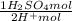`(1H_(2)SO_4 mol)/(2H^(+) mol)` = 8,96x10⁻³ moles of H₂SO₄

These moles comes from:

8,96x10⁻³ moles of H₂SO₄ ×
`(98,1 g)/(1 mol)` ×
`(100 gSolution)/(12 gH_(2)SO_4 )` ×
`(1 mL)/(1,080 g)` =

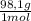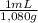6,78 mL of 12,0wt% H₂SO₄

I hope it helps!