Question

Find the distance between lines 8x−15y+5=0 and 16x−30y−12=0.

Answer 1

The distance between the two parallel lines is 11/17 units

Explanation:

we have

8x-15y+5=0 -----> equation A

16x-30y-12=0 ----> equation B

Divide by 2 both sides equation B

8x-15y-6=0 ----> equation C

Compare equation A and equation C

Line A and Line C are parallel lines with different y-intercept

step 1

Find the slope of the parallel lines (The slope of two parallel lines is the same)

8x-15y+5=0

15y=8x+5

`y=(8)/(15)x+(1)/(3)`

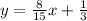
the slope is

`m=(8)/(15)`

step 2

Find the slope of a line perpendicular to the given lines

Remember that

If two lines are perpendicular then their slopes are opposite reciprocal (the product of their slopes is -1)

`m1*m2=-1`

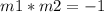
we have

`m1=(8)/(15)`

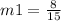
therefore

`m2=-(15)/(8)`

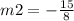
step 3

Find the equation of the line perpendicular to the given lines

assume any point that lie on line A

`y=(8)/(15)x+(1)/(3)`

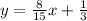
For
`x=0`

`y=(1)/(3)`

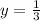
To find the equation of the line we have

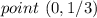`point\ (0,1/3)` ---> is the y-intercept

`m=-(15)/(8)`

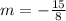
The equation in slope intercept form is

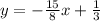`y=-(15)/(8)x+(1)/(3)` -----> equation D

step 4

Find the intersection point of the perpendicular line with the Line C

we have the system of equations

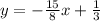`y=-(15)/(8)x+(1)/(3)` ----> equation D

`8x-15y-6=0` ---->
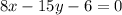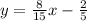`y=(8)/(15)x-(2)/(5)` ----> equation E

equate equation D and equation E and solve for x

`(8)/(15)x-(2)/(5)=-(15)/(8)x+(1)/(3)`

`(8)/(15)x+(15)/(8)x=(1)/(3)+(2)/(5)`

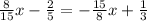
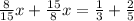
Multiply by 120 both sides to remove fractions

`64x+225x=40+48`

`289x=88`

`x=88/289`

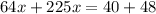
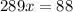
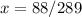
Find the value of y

`y=-(15)/(8)(88/289)+(1)/(3)`

`y=-(206)/(867)`

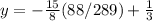
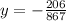
the intersection point is
`((88)/(289),-(206)/(867))`

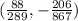
step 5

Find the distance between the points
`(0,(1)/(3))` and
`((88)/(289),-(206)/(867))`

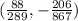
the formula to calculate the distance between two points is equal to

`d=\sqrt{(y2-y1)^(2)+(x2-x1)^(2)}`

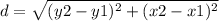
substitute the values

`d=\sqrt{(-(206)/(867)-(1)/(3))^(2)+((88)/(289)-0)^(2)}`

`d=\sqrt{(-(495)/(867))^(2)+((88)/(289))^(2)}`

`d=\sqrt{((245,025)/(751,689))+((7,744)/(83,521))}`

`d=\sqrt{(314,721)/(751,689)}`

`d=(561)/(867)\ units`

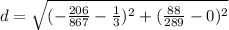
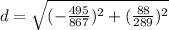
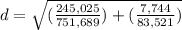
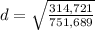
Simplify

`d=(11)/(17)\ units`

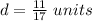
therefore

The distance between the two parallel lines is 11/17 units

see the attached figure to better understand the problem