Question

Bag A contains 3 whites marbles and 2 red marbles . bag B contains 6 white marbles and 3 red marbles . a person draws one marble from each bag find the probability that both marbles are white

Answer 1

Probability of drawing two white marbles from each bag is 2/5.

Solution:

Consider A as first bag and B as second bag; Probability of drawing one white marble from A and B are P (A) and P (B) respectively

`P(A)=\frac{\text {number of white marbles in } A}{\text {total number of marbles in } A}=(3)/(5)`

`P(B)=\frac{\text {number of white marbles in } B}{\text {total number of marbles in } B}=(6)/(9)`

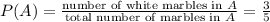
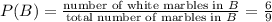
Since they are independent events, multiply both the probability of the events to find the total probability

`\text { Total probability }=P(A) * P(B)=(3)/(5) * (6)/(9)=(2)/(5)`

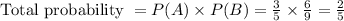
Hence, the probability is 2/5