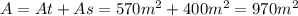Question

Find the area of this irregular polygon.

Answer 1

970m^{2}

Explanation:

This polygon can be divided in two figures: one is a triangle, an the other one is a square.

We'll begin calculating the triangle's area, using the following formula:

`At= (b.h)/(2)`

Where:

`H= height = 30 m`

`B = 9 m + 9 m + 20 m = 38 m`

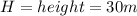
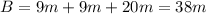
As you can see, I added both sides of the triangle that measure 9 m and also the lenght of the square that measures 20 m! This added up is what the base of the triangle measures on total.

`At= (38 m .30 m)/(2)`

`At= (1140 m^(2) )/(2)`

`At= 570 m^(2)`

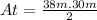
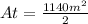
Now we are going to calculate the square's area, that is much more simple:

`As= L^(2)`

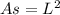
Where:

`L=20 m`

`As= (20 m)^(2) = 400m^(2)`

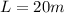
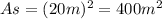
To know the whole figure's area, we add up both areas:

`A = At+As = 570m^(2) +400m^(2)=970m^(2)`