Question

The slopeof a linear function h(x) is 2. Suppose the function is translated 8 units up to get d(x). How can h(x) be translated to the left or right to represent the same function d(x)?

Answer 1

4 units to the right

Explanation:

Since all we know about function h(x) is that it has slope 2, we don't know its y intercept, so let's call it b and write the equation of that first line as:

`h(x) = 2x + b`

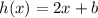
Now, we translated that function up 8 units to get function d(x). This vertical displacement is expressed by adding 8 units to function h(x):

`d(x) = h(x) + 8 = 2x+b+8`

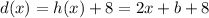
We want to translate this last function d(x) either to the right or to the left in order to get the same expression as for function h(x).

Recall that translations to the right or left a fix number of units "c" affects the "x" coordinate of the function (by adding "c", we move to the left c units the graph of the function, and by subtracting "c" we move to the right the graph of the function).

So represent a generic shift for d(x) for example to the right in c units:

`d(x) = 2(x-c)+x^(2) +b+8= 2x-2c+b+8`

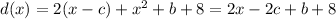
Now we want this new shifted function to be EXACTLY as h(x) so we write the equality:

`2x-2c+8+b=h(x)=2x+b\\2x-2c+8+b = 2x+b`

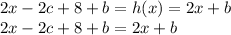
We can solve in the equation for the value "c" we need for the translation:

`2x-2c+8+b = 2x+b\\-2c+8=0\\2c=8\\c=(8)/(2) = 4`

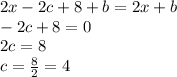
subtracting 2x from both sides, and also subtracting "b" from both sides.

This means that the translation to the right by 4 units will result on the exact same graph as for function h(x)