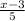Answer: The simplified value of the given expression will be
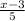
Explanation:
The expression of the given statement becomes:
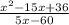
To solve the above expression, we need to factorize the quadratic equation by middle term splitting:

Omitting out the factor '(x - 12)' from denominator and numerator, we get:
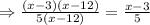
Hence, the simplified value of the given expression will be