Question

A science student riding on a flatcar of a train moving at a constant speed of 11.2 m/s throws a ball toward the caboose along a path that the student judges as making an initial angle of 51° to the horizontal. The teacher, who is standing on the ground nearby, observes the ball rising vertically. How high does the ball rise?

Answer 1

ball rise height is 19.34 m

Step-by-step explanation:

given data

speed = 11.2 m/s

angle = 51°

to find out

How high does the ball rise

solution

we take here velocity vector as x and y component in horizontal and vertical direction

velocity (x) = v cos 51

velocity (y) = v sin 51

and ratio between these component is

`(Vy)/(Vx) =(vsin60)/(vcos60)`

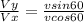
Vy = tan51 Vx

Vy = 1.23 Vx

we consider here speed v is relative to teacher

and here no motion in x refer to teacher so V will be 0

so equation of motion

V = Vx + v = 0

Vx = -v

Vx = - 11.2 m/s

so ball thrown backward student ref is

Vref y = Vy

Vref y = 1.23 Vx

Vref y = 1.23 (-11.2)

Vref y = 13.77 m/s

and

height of ball by kinematic equation

height =
`(v^2)/(2g)`

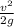
here v is 13.77 and g = 9.8

height =
`(13.77^2)/(2(9.8))`

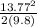
height = 19.34 m

so ball rise height is 19.34 m