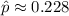Answer:
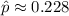
Explanation:
Given : This exercise provides information about data in a survey of students.
Of the students who identified their class year in the survey, 82 were first years, 219 we're sophomores, 28 we're juniors, and 61 were seniors.
Total students sampled =
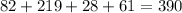
Number of upper class students (juniors or seniors.) =
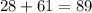
Now, the proportion who are upper class students (juniors or seniors.) will be :-
![\hat{p}=\frac{\text{Number of upper class students}}{\text{Total students}}\\\\=(89)/(390)=0.228205128205\\\\\approx0.228\ \ [ \text{Round to three decimal places.}]](https://img.qammunity.org/2020/formulas/mathematics/college/o1sifk55sbd6n7rgdcyft5wqsgrsd4v3e5.png)
Hence,