Question

(1 point) A tank contains 23402340 L of pure water. Solution that contains 0.050.05 kg of sugar per liter enters the tank at the rate 55 L/min, and is thoroughly mixed into it. The new solution drains out of the tank at the same rate. (a) How much sugar is in the tank at the begining? y(0)=y(0)= equation editorEquation Editor (kg) (b) Find the amount of sugar after t minutes. y(t)=y(t)= equation editorEquation Editor (kg) (c) As t becomes large, what value is y(t)y(t) approaching ? In other words, calculate the following limit. limt→[infinity]y(t)=limt→[infinity]y(t)= equation editorEquation Editor (kg)

Answer 1

Let
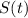`S(t)` be the amount of sugar in the tank at time
`t`.

a. The tank contains only pure water at the start, so
`\boxed{S(0)=0}`.

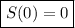b. The inflow rate of sugar is

`{S_(\rm in)}'=\left(0.05(\rm kg)/(\rm L)\right)\left(5(\rm L)/(\rm min)\right)=\frac1{40}(\rm kg)/(\rm min)`

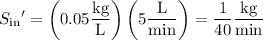
and the outflow rate is

`{S_(\rm out)}'=\left(\frac S{2340}(\rm kg)/(\rm L)\right)\left(5(\rm L)/(\rm min)\right)=\frac S{468}(\rm kg)/(\rm min)`

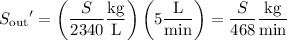
so the net rate at which
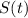`S(t)` changes over time is governed by

`S'=\frac1{40}-\frac S{468}\implies S'+\frac S{468}=\frac1{40}`

Multiply both sides by
`e^(t/468)`,

`e^(t/468)S'+(e^(t/468))/(468)S=(e^(t/468))/(40)`

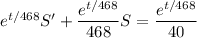
and condense the left side as the derivative of a product,

`\left(e^(t/468)S\right)'=(e^(t/468))/(40)`

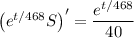
Integrate both sides to get

`e^(t/468)S=(117e^(t/468))/(10)+C`

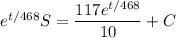
and solve for
`S`:

`S=(117)/(10)+Ce^(-t/468)`

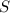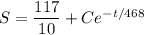
With
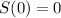`S(0)=0`, we find
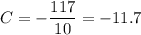`C=-(117)/(10)=-11.7`, so that

`\boxed{S(t)=11.7-11.7e^(-t/468)}`

c. As
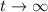`t\to\infty`, the exponential term will converge to 0, leaving a fixed amount of 11.7 kg of sugar in the solution.