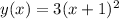Answer:
Shift 1 units towards left and then stretch vertically 3 times.
Explanation:
We are given that a parent function
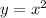
After transformation, we get a graph
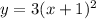
We have to find that how the parent function transformed to produce the graph of
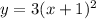
The parent graph is shifted 1 unit towards left by the rule of transformation
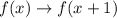
By applying this rule then, we get
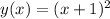
Now, the parent function stretched vertically 3 times the previous graph by the rule

Apply this rule then, we get