Question

The odds of an individual getting struck by lightening in one year are roughly 1 in a million; for this question assume this probability is exactly that ratio: 10−6 . In a randomly chosen set of eleven people, what are the odds that exactly 2 of them are hit by lightening in a given year? What is the correct probability distribution to use to answer this question? Why is this the correct choice of probability distribution?

Answer 1

There is a
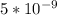`5*10^(-9)`% probability that exactly 2 of them are hit by lightening in a given year

The correct probability distribution to solve this problem is the binomial probability.

This is exact the type of problem that the binomial probability is used, when we want to find the probability of a exact number of events happening in a number of trials.

Explanation:

Here we use the binomial probability.

For the binomial probability calculus we have these following parameters.

`n:` The number of repeated trials

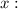`x:` The desired number of sucesses.

`p:` The probability of success on each individual trial.

The probability of exactly x sucesses on n repeated trials is given by:

`P = C_(n,x).p^(x).(1 - p)^(n-x)`

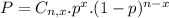
In which
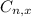`C_(n,x)` is the combination of the number of repeated trials and the desired number of sucesses. The combination formula is given by:

`C_(n,x) = (n!)/(x!(n-x)!)`

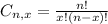
Here we have:

Sample of eleven people, so
`n = 11`

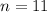
Exactly two of them being hit, so
`x = 2`

`p = 10^(-6)`

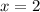
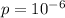
The probability that exactly two people are hit by a lightning is:

`P = C_(n,x).p^(x).(1 - p)^(n-x)`

`P = C_(11,2).(10^(-6))^(2).(1 - 10^(-6))^(9)`

`P = 55.(10^(-6))^(2).(1 - 10^(-6))^(9)`

`P = 5*10^(-11)`

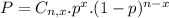
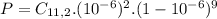
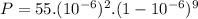
Multiplying by 100, to write in percent notation.

There is a
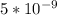`5*10^(-9)`% probability that exactly 2 of them are hit by lightening in a given year