Question

Two brothers, Kevin and Charles, each inherit $49000. Kevin invests his inheritance in a savings account with an annual return of 1.9%, while Charles invests his inheritance in a CD paying 5.6% annually. How much more money than Kevin does Charles have after 1 year?

Answer 1

$ 1813

Explanation:

Given,

Investment of Kevin,
`P_1` = $ 49000,

Rate of interest = 1.9 % annually,

Thus, his interest,
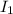`I_1` = 1.9% of 49000

`=(1.9* 49000)/(100)`

`=(93100)/(100)`

`=\$ 931`

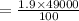
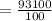
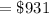
Hence, Kelvin's amount after 1 year,

`A_1=P_1+I_1=49000+931=\$ 49931`

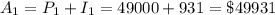
Now, Investment of Charles,
`P_2` = $ 49000,

Rate of interest = 5.6 % annually,

Thus, his interest,
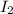`I_2` = 5.6% of 49000

`=(5.6* 49000 )/(100)`

`=(274400)/(100)`

`=\$ 2744`

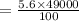
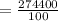
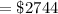
Hence, Kelvin's amount after 1 year,

`A_2=P_2+I_1=49000+2744=\$ 51744`

`\because A_2-A_1=51744-49931 = \$ 1813`

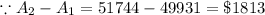
Therefore, after 1 year Kevin has $1813 more than Charles.