Question

If 140. mg of fluorine-18 is shipped at 8:00 A.M., how many milligrams of the radioisotope are still active when the sample arrives at the radiology laboratory at 1:30 P.M.

Answer 1

17.4 mg of the radioisotope are still active when the sample arrives at the radiology laboratory at 1:30 P.M.

Step-by-step explanation:

Half-life of F-18 is found to be 109.7 minutes

Rate constant

`$k=\frac{0.693}{t_{(1)/(2)}}=0.00632$`

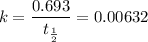
t = time taken = 5 hours 30 minutes = 330 minutes

`$\ln [A]=\ln [A]_(0)-k t$`

[A] is the final quantity

`[A]_0` is the initial quantity

Plugging the values and solving for [A]

`\\$\ln [A]=\ln (140 m g)-\left(0.00632 \min ^(-1) * 330 \min \right)$\\\\$\ln [A]=4.942-2.085$\\\\$\ln [A]=2.857$\\\\$[A]=e^(2.857)$`

[A] = 17.4mg is the Answer

Answer 2

To answer the question we need to know the half-life of fluorine for reference we have chlorine half-life as 110. The fraction of chlorine remaining would be given by
`0.5^n`

Step-by-step explanation:

Here we know and is known as the number of Half-life that have elapsed during this process Now we are giving the time of start as 8 a.m. and time of finishing at 1:30 p.m., so the time between is 5 ½ hours. Which on converting in minutes will give 330 minutes.

So Half Life elapsed would be given by =
`330/110=3`

Hence the amount remaining would be =
`0.5 ^3 * 125 =15.625`