Question

I need to know then measurement of c

Answer 1

`\huge{ \boxed{ \bold{ \sf{ \pink{80°}}}}}`

Explanation:

✰
`\underline{ \sf{First, \: Let's \: learn \: about \: internal \: angles \: of \: a \: triangle}}`

The angles which are formed inside by the joining of arms of a triangle is called interior angles of a triangle. According to the figure provided , In ∆ ABC , ∠ABC , ∠ ACB , ∠ BAC are interior angles because they lie inside the ∆ ABC and sum of such interior angles is always 180°.

❀
`\sf{Now, \: Let's \: solve} :`

☄
`\underline{ \underline{ \sf{Provided : }}}`

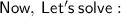
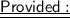
• ∠B = 3x°
• ∠ C = 4x°
• ∠ A = 2x°

☄
`\underline{ \sf{To \: Find :}}`

• Measure of C

✏
`\text{Set \: up \: an \: equation \: and \: solve \: for \: x.}`

`\text{{∠a \: + \: ∠ \: b \: + \: ∠ \: c \: = \: 180 \degree}}`

→
`\sf{2x + 3x + 4x = 180 \degree}`

→
`\sf{9x = 180 \degree}`

→
`\sf{ (9x)/(9) = (180 \degree )/(9) }`

→
`\sf{x = 20 \degree}`

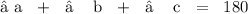
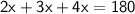
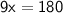
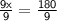
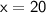
The value of x is 20°. As we are asked to find the measure of ∠C , substituting the value of x in 4x :

`\text{∠c = 4x}`

→
`\sf{∠ \: c \: = 4 * 20 \degree}`

→
`\boxed{ \bold{ \pink{ \sf{∠c \: = 80 \degree}}}}`

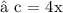
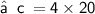
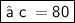
Hence , The measure of ∠ C is 80 °

Hope I helped ! ッ

Have a wonderful day / night ! ♡

▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁

Answer 2

C is 666666688888 from my lookings. Jk