Question

If the spacing between the lines is 2000 nm, and the angle of the second-order ray is 48.5°, what is the wavelength of the light? a. 1500 nm b. 1000 nm c, 750 nrn d. 2000 nnm e. We can't determine the wavelength from the information.

Answer 1

Wavelength of the light is 750 nm.

Step-by-step explanation:

Given that,

Spacing between the lines,
`d=2000\ nm=2* 10^(-6)\ m`

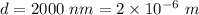
Order of the grating, n = 2

The second order is formed at an angle of 48.5°. The equation of diffraction grating is given by :

`d\ sin\theta=n\lambda`

`\lambda=(d\ sin\theta)/(n)`

`\lambda=(2* 10^(-6)* sin(48.5))/(2)`

`\lambda=7.48* 10^(-7)\ m`

`\lambda=748\ nm`

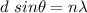
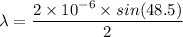
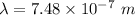
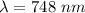
or

`\lambda=750\ nm`

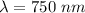
So, the wavelength of the light is 750 nm. Hence, this is the required solution.