Question

A firm is accustomed to training operators who do certain tasks on a production line. Those operators who attend the training course are known to be able to meet their production quotas 90% of the time. New operators who do not take the training course only meet their quotas 65% of the time. Fifty percent of new operators attend the course. Given that a new operator meets her production quota, what is the probability that she attended the program?

Answer 1

Probability = 0.58

Explanation:

This problem is solve by using Baye's Probability.

Let P(A) = Probability that operator attended training course = 50% = 0.5

P(B) = Probability that operator not attended training course = 50% = 0.5

Also P(Q) = Probability that operator meet their production quotas

Then, P(Q|A) = 90% = 0.9

P(Q|B) = 65% = 0.65

P(A|Q) = ?

Then by Baye's Theorem,

`P(A|Q) = (P(Q|A) * P(A))/(P(Q|A) * P(A)+P(Q|B) * P(B))`

⇒
`P(A|Q) = (0.9 *\0.5 )/(0.9 *\0.5+0.65 *\0.5)`

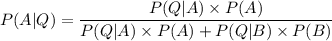
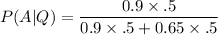
⇒ P(A|Q) = 0.58

which is required probability.