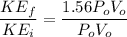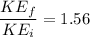Question

(a) An ideal gas initially at pressure p0 undergoes a free expansion until its volume is 3.80 times its initial volume. What then is the ratio of its pressure to p0? (b) The gas is next slowly and adiabatically compressed back to its original volume. The pressure after compression is (3.80)1/3p0. Is the gas monatomic, diatomic, or polyatomic? (c) What is the ratio of the average kinetic energy per molecule in this final state to that in the initial state?

Answer 1

a)P/Po=0.263

b)γ=1.33 So gas is triatomic

c)
`(KE_f)/(KE_i)=1.56`

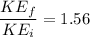
Step-by-step explanation:

a)

initial pressure = Po

Initial volume = Vo

Final volume = 3.8 Vo

Lets take final pressure is P

we know that for free expansion process

PV= Constant

Po x Vo = P x 3.8 Vo

P=0.263 Po

So

P/Po=0.263

b)

Now gas is compressed in adiabatic manner

Final pressure = 1.56 Po

=1.56 Po

We know that for adiabatic process

`P_1V_1^(\gamma)=P_2V_2^(\gamma)`

`(V_2)/(V_1)=\left((P_1)/(P_2)\right)^{(1)/(\gamma)}`

`0.263P_o(3.8V_o)^(\gamma)=1.56P_o* V_o^(\gamma)`

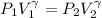
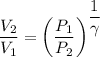
γ=1.33 So gas is triatomic

c)

We know that average kinetic energy given as

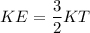
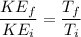`KE=(3)/(2)KT`

`(KE_f)/(KE_i)=(T_f)/(T_i)`\

`(KE_f)/(KE_i)=(P_fV_f)/(P_iV_i)`

`(KE_f)/(KE_i)=(1.56P_oV_o)/(P_oV_o)`

`(KE_f)/(KE_i)=1.56`